14. Climbing Flight
Climbing Flight
Now let's consider climbing flight where the flight path angle is positive (\gamma > 0).
We'll assume we're climbing at constant velocity, so the forces and moments balance to zero. We'll also assume zero angle of attack for now.
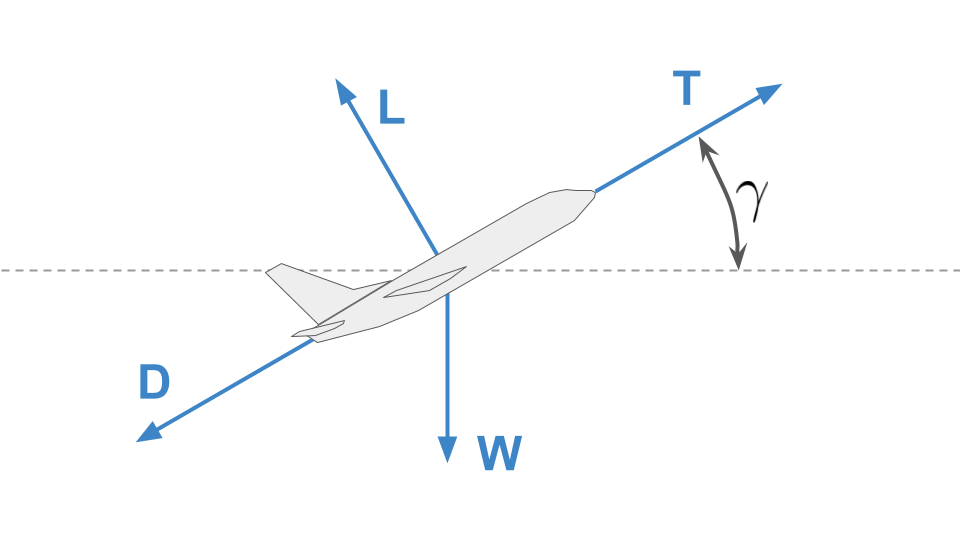
Working in the body frame, we can see that the thrust is balanced by the drag plus a component of the weight:
\begin{aligned}
T &= D + W \sin \gamma \\ \\
\sin \gamma &= \frac{T-D}{W}
\end{aligned}
We can calculate the vertical velocity \dot{z} as
\begin{aligned}
\dot{z} &= V \sin \gamma \\ \\
\dot{z} &= \frac{V(T-D)}{W}
\end{aligned}
And the lift is balanced by a portion of the weight:
\begin{aligned}
L &= W \cos \gamma \\ \\
C_L \bar{q} S &= W \cos \gamma
\end{aligned}
We could solve this for coefficient of lift and then for angle of attack.